32 Also take your flocks and your herds, as ye have said, and be gone; and bless me also. –Exodus 12, KJV
A Bug in My Ass
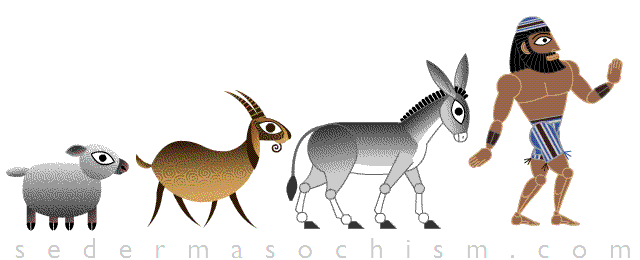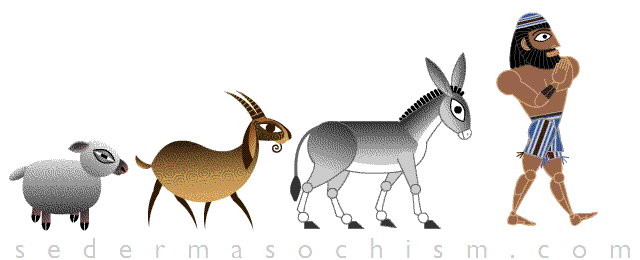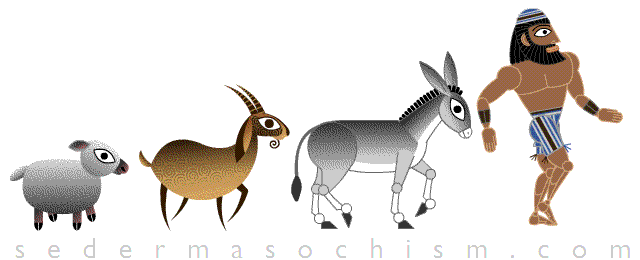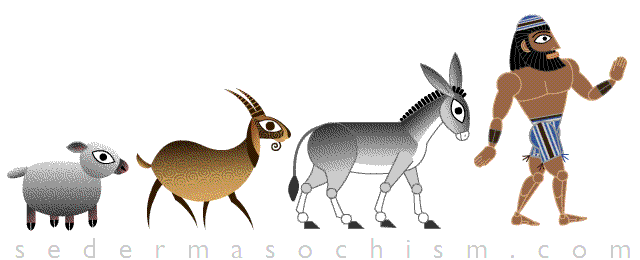
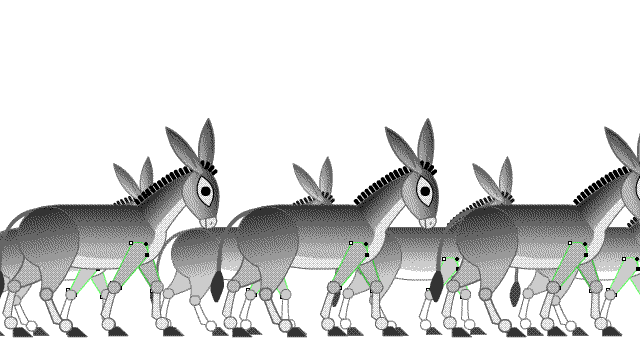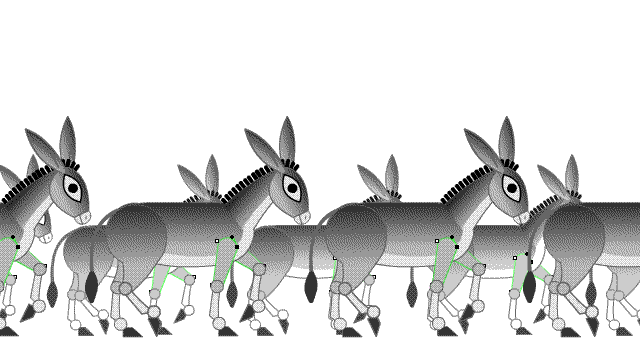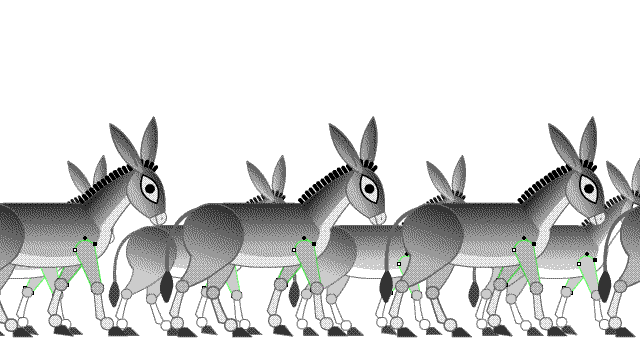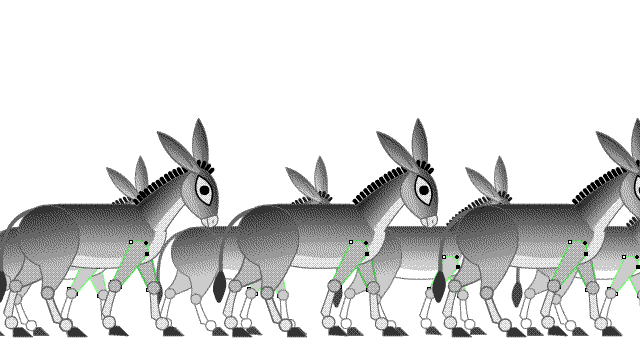
So I’m animating an ass for Seder-Masochism, so the Hebrews have something to carry their stuff out of Egypt so their arms are free to let them walk jauntily and leap around. I export a test and see this weird artifact on the upper foreleg. Flash is rendering the shape as it looks in vector editing mode. If you look carefully you’ll see the point on the upper right has a little bezier handle. Weird, right?
It’s a bug in Flash (pre-Adobe Macromedia Flash 8, the only Flash I use), the likes of which I’ve never seen before. To make the artifact go away I had to re-draw the foreleg. Below is a render sans bug.
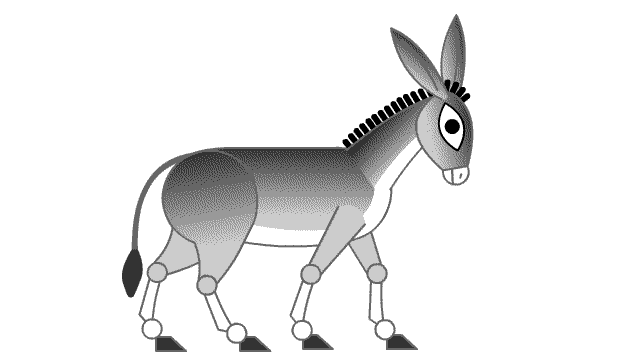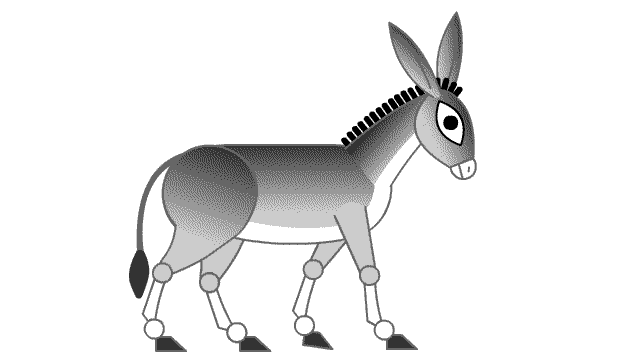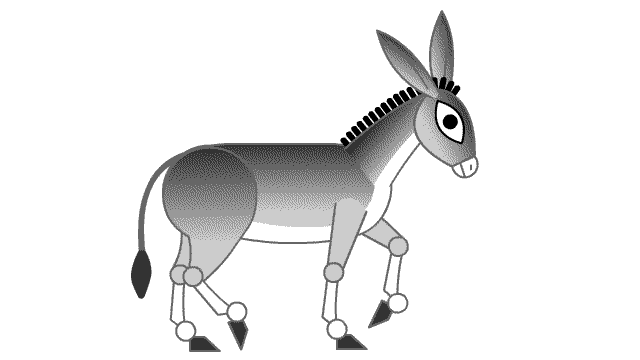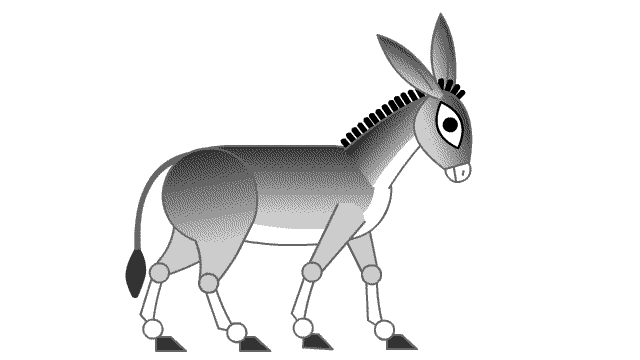 What’s great about this is it solved my problem about how to best use “ass” in a blog post title. I was considering “What an Ass” or “A Fine Ass” or “I Made Myself an Ass,” but this is much better.
What’s great about this is it solved my problem about how to best use “ass” in a blog post title. I was considering “What an Ass” or “A Fine Ass” or “I Made Myself an Ass,” but this is much better.
Crossing the Red Sea
Gettin’ pumped to conquer the Promised Land!
Every country that exists originated through violent conquest. The victims of these conquests were victors and conquerors earlier. Is violent conquest legitimate? If not, then no country or nation is legitimate. If nations are legitimate, then violent conquest must be also. I ask these questions as I animate the mythical Ancient Hebrews’ quest to conquer Canaan (which was already occupied). There’s just no way to make it look good by modern humanitarian values, and I wonder why that is.
Happy Heeb
This Hebrew is getting ready to walk right out of Egypt, bouncing along to a song that has beats every 19 frames (oy!). Here’s how he looked in an earlier scene.
Parted Sea Waves
Moses parts the Red Sea
There are better ways to encode animated gifs, but I don’t have access to them right now. This was exported from Macromedia Flash 8 (2005), so it’s dithered. If anyone knows of a safe, modern online .mov-to-.gif converter that uses a non-dithering encoder, or same to download for my antique (OS 10.6) Mac, let me know.